数据结构与算法/leetcode/lintcode题解
未点赞
未收藏
github
纠错
贡献更多优质内容

Bipartial Graph - Part I - 二分图一•二分图判定

Question

Problem Statement

时间限制:10000ms

单点时限:1000ms

内存限制:256MB

描述

大家好，我是小Hi和小Ho的小伙伴Nettle，从这个星期开始由我来完成我们的Weekly。

新年回家，又到了一年一度大龄剩男剩女的相亲时间。Nettle去姑姑家玩的时候看到了一张姑姑写的相亲情况表，上面都是姑姑介绍相亲的剩男剩女们。每行有2个名字， 表示这两个人有一场相亲。由于姑姑年龄比较大了记性不是太好，加上相亲的人很多，所以姑姑一时也想不起来其中有些人的性别。因此她拜托我检查一下相亲表里面有没有错误 的记录，即是否把两个同性安排了相亲。

OK，让我们愉快的暴力搜索吧！

才怪咧。

对于拿到的相亲情况表，我们不妨将其转化成一个图。将每一个人作为一个点**(编号1..N)**，若两个人之间有一场相亲，则在对应的点之间连接一条无向边。(如下图)

img1

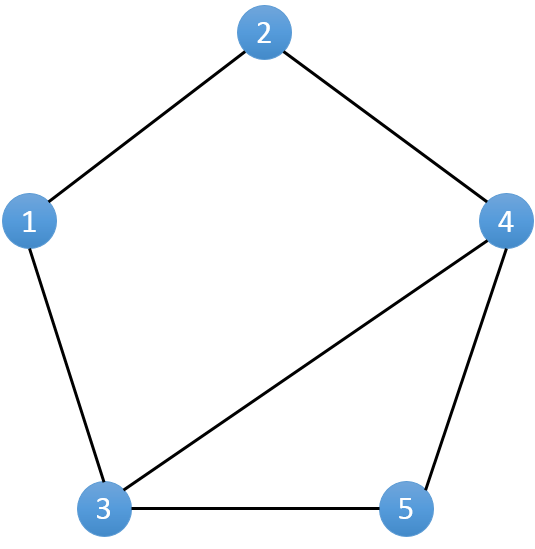
因为相亲总是在男女之间进行的，所以每一条边的两边对应的人总是不同性别。假设表示男性的节点染成白色，女性的节点染色黑色。对于得到的无向图来说，即每一条边的两端 一定是一白一黑。如果存在一条边两端同为白色或者黑色，则表示这一条边所表示的记录有误。

由于我们并不知道每个人的性别，我们的问题就转化为判定是否存在一个合理的染色方案，使得我们所建立的无向图满足每一条边两端的顶点颜色都不相同。

那么，我们不妨将所有的点初始为未染色的状态。随机选择一个点，将其染成白色。再以它为起点，将所有相邻的点染成黑色。再以这些黑色的点为起点，将所有与其相邻未染色 的点染成白色。不断重复直到整个图都染色完成。(如下图)

img2

在染色的过程中，我们应该怎样发现错误的记录呢？相信你一定发现了吧。对于一个已经染色的点，如果存在一个与它相邻的已染色点和它的颜色相同，那么就一定存在一条错误 的记录。(如上图的4，5节点)

到此我们就得到了整个图的算法：

  1. 选取一个未染色的点u进行染色
  2. 遍历u的相邻节点v：若v未染色，则染色成与u不同的颜色，并对v重复第2步；若v已经染色，如果 u和v颜色相同，判定不可行退出遍历。
  3. 若所有节点均已染色，则判定可行。

接下来就动手写写吧！

输入

第1行：1个正整数T(1≤T≤10)

接下来T组数据，每组数据按照以下格式给出：

第1行：2个正整数N,M(1≤N≤10,000，1≤M≤40,000)

第2..M+1行：每行两个整数u,v表示u和v之间有一条边

输出

第1..T行：第i行表示第i组数据是否有误。如果是正确的数据输出”Correct”，否则输出”Wrong”

样例输入

2
5 5
1 2
1 3
3 4
5 2
1 5
5 5
1 2
1 3
3 4
5 2
3 5

copy

样例输出

Wrong
Correct

copy

题解

二分图中最简单的题，思路原文中已提到，这里就不赘述了，简单实现的话可以使用二维数组，如果要模拟图的操作的话可以自定义类。

Java

import java.util.*;
import java.util.Queue;

class UndirectedGraphNode {
    int label;
    int color;
    ArrayList<UndirectedGraphNode> neighbors;
    UndirectedGraphNode(int x) {
        this.label = x;
        this.color = 0;
        this.neighbors = new ArrayList<UndirectedGraphNode>();
    }
}

public class Main {
    public static void main(String[] args) {
        Scanner in = new Scanner(System.in);
        int T = in.nextInt();
        for (int i = 1; i <= T; i++) {
            int N = in.nextInt();
            int M = in.nextInt();
            // initialize graph
            List<UndirectedGraphNode> graph = new ArrayList<UndirectedGraphNode>();
            for (int n = 1; n <= N; n++) {
                graph.add(new UndirectedGraphNode(n));
            }
            // construct graph
            for (int j = 1; j <= M; j++) {
                int u = in.nextInt(), v = in.nextInt();
                graph.get(u - 1).neighbors.add(graph.get(v - 1));
                graph.get(v - 1).neighbors.add(graph.get(u - 1));
            }
            // solve
            if (solve(graph)) {
                System.out.println("Correct");
            } else {
                System.out.println("Wrong");
            }
        }
    }

    public static boolean solve(List<UndirectedGraphNode> graph) {
        // 1 for white, -1 for black, 0 for uncolored
        for (UndirectedGraphNode node : graph) {
            if (node.color == 0) {
                node.color = 1;
                Queue<UndirectedGraphNode> q = new LinkedList<UndirectedGraphNode>();
                q.offer(node);
                while (!q.isEmpty()) {
                    int qSize = q.size();
                    for (int i = 0; i < qSize; i++) {
                        UndirectedGraphNode qNode = q.poll();
                        for (UndirectedGraphNode neighbor : qNode.neighbors) {
                            if (neighbor.color == 0) {
                                neighbor.color = -1 * qNode.color;
                                q.offer(neighbor);
                            } else if (neighbor.color + qNode.color != 0) {
                                // the color of qNode is the same with neighbor
                                return false;
                            }
                        }
                    }
                }
            }
        }

        return true;
    }
}

copy

源码分析

使用 BFS 不容易爆栈。

复杂度分析

时间复杂度 O(V+E)O(V + E).